
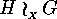 , where
, where  is
a group acting on
is
a group acting on 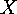 while
while 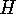 acts on
acts on 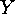 . Now we
consider the particular case where
. Now we
consider the particular case where  is a permutation group, say
is a permutation group, say
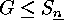 , and where we take for
, and where we take for  the natural action of
the natural action of  on
on 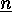 .
In this case we shorten the notation by putting
.
In this case we shorten the notation by putting

A particular case is 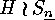 , the complete monomial group
of degree
, the complete monomial group
of degree 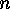 over
over 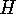 . Many important groups are of this form, examples will
be given in a moment. In the case when
. Many important groups are of this form, examples will
be given in a moment. In the case when
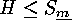 , then
, then 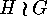 has the following natural embedding
into
has the following natural embedding
into  :
:
This can be seen as follows:
Remember the direct factors 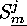 , for
, for 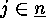 , of the base
group
, of the base
group 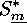 of
of 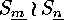 (cf. the remark on
(cf. the remark on 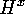 in
in ![]() ). Its image
). Its image 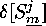 acts on the block
acts on the block
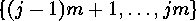 as
as  does on
does on 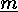 , while the image
, while the image  of the complement
of the complement 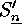 of the base group acts on the set of
these
of the base group acts on the set of
these 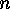 subsections
subsections 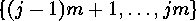 of length
of length 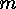 of the set
of the set 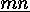 as
as 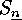 does act on
does act on 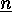 . For example the
element
. For example the
element
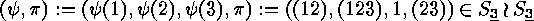
is mapped under 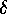 onto
onto
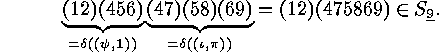
The image of 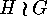 under
under 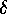 will be denoted as follows:
will be denoted as follows:
It is called the plethysm
of  and
and 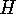 , for reasons which will become clear later.
, for reasons which will become clear later.