In this section we investigate some necessary conditions that have to hold if
there exists a ![]() -
-![]() design with automorphism group
design with automorphism group ![]() .
So we throughout assume
.
So we throughout assume ![]() to be a point set of
to be a point set of ![]() points. A
points. A ![]() -set
simply means a subset of size
-set
simply means a subset of size ![]() of
of ![]() .
.
We start using only some divisibility conditions.
Theorem 1:
If ![]() -
-![]() is an admissible parameter set for a
is an admissible parameter set for a ![]() -design then
-design then ![]() is
even. If in addition
is
even. If in addition
![]() for some prime
for some prime ![]() then
3 divides
then
3 divides ![]() and 5 does not divide
and 5 does not divide ![]() .
.
Proof
By double counting the number ![]() of blocks of a
of blocks of a ![]() -
-![]() design is always
design is always

Because a ![]() -design is also a
-design is also a ![]() -design with the same
number of blocks the corresponding formula for
-design with the same
number of blocks the corresponding formula for ![]() yields
the
yields
the ![]() .
In case
.
In case ![]() and
and ![]() then
then
![]() such that
such that ![]() is even.
is even.
Continuing with ![]() in the same way then
in the same way then
![]() such that
if
such that
if ![]() then 3 does not divide
then 3 does not divide ![]() and hence has to divide
and hence has to divide ![]() The case
The case ![]() is clearly impossible.
is clearly impossible.
Continuing in this way we obtain from the
formula for ![]() that 5 is a divisor of
that 5 is a divisor of
![]() such that 5 does not divide
such that 5 does not divide ![]() .
.
![]()
We get some sharper results if we assume ![]() as a group
of automorphisms. Our main tool will be to analyse orbits of
as a group
of automorphisms. Our main tool will be to analyse orbits of
![]() on subsets
on subsets ![]() of the point set
of the point set ![]() by looking at the
set stabilizer
by looking at the
set stabilizer
![]() of such a subset.
of such a subset.
We introduce some general notations. We assume that a finite group ![]() acts on a finite set
acts on a finite set ![]() of
of ![]() points. For a subset
points. For a subset ![]() of
of ![]() the length of the orbit
the length of the orbit ![]() under
under ![]() is
is

Orbits on ![]() -sets
-sets ![]() and
and ![]() -sets
-sets ![]() for
for
![]() are related by some numbers which are important for the
construction of
are related by some numbers which are important for the
construction of ![]() -designs.
-designs.
We define
Alltop's Lemma 2:
Let a group ![]() act on a set
act on a set ![]() and let
and let ![]() be a
be a ![]() -set and
-set and ![]() a
a ![]() -set of
-set of ![]() . Then
. Then
This follows easily by doubly counting
The group ![]() acts
acts ![]() -homogeneously if it is transitive on the
-homogeneously if it is transitive on the ![]() -sets.
-sets.
Corollary 3:
Let ![]() act
act ![]() -homogeneously in Alltop's Lemma. Then
-homogeneously in Alltop's Lemma. Then
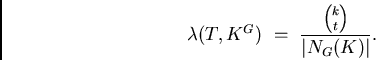
Proof.
Substituting
![]() ,
,
![]() , and
, and
![]() in the equation and cancelling
in the equation and cancelling ![]() yields
the claimed result.
yields
the claimed result.
![]()
In the special case of ![]() and
and
![]() we obtain that
we obtain that
![]() divides 10.
In particular, if 5 does not divide
divides 10.
In particular, if 5 does not divide ![]() this implies
that
this implies
that
![]() divides 2.
divides 2.
We will construct orbit representatives by constructing ![]() -sets invariant under a
prescribed subgroup
-sets invariant under a
prescribed subgroup ![]() . From these we first have to remove those invariant under a
larger group and then have to decide whether they lie in the same orbit.
This can be done using the next Lemma [15].
. From these we first have to remove those invariant under a
larger group and then have to decide whether they lie in the same orbit.
This can be done using the next Lemma [15].
Lemma 4
Let a group ![]() act on a set
act on a set ![]() and let
and let
![]() be two t-sets
having the same stabilizer
be two t-sets
having the same stabilizer ![]() in
in ![]() . Let
. Let ![]() for some
for some ![]() .
Then
.
Then ![]() .
.
Proof.
We have
If 5 divides
![]() then 5 divides
then 5 divides ![]() by Theorem 1. We know from group theory [10] that
then there exists only one conjugacy class of subgroups of order
by Theorem 1. We know from group theory [10] that
then there exists only one conjugacy class of subgroups of order ![]() in
in ![]() . Such a subgroup is a dihedral group
. Such a subgroup is a dihedral group ![]() with a cyclic normal subgroup of
order
with a cyclic normal subgroup of
order ![]() . A subgroup
. A subgroup ![]() of order 5 of
of order 5 of ![]() then has orbits of length
5 and 1 only and
then has orbits of length
5 and 1 only and
![]() .
The
.
The ![]() orbits of length 5 form one orbit of
orbits of length 5 form one orbit of ![]() on 5-element subsets.
Thus, by Lemma 4, there is only one orbit of
on 5-element subsets.
Thus, by Lemma 4, there is only one orbit of ![]() on 5-sets with a stabilizer of order 5.
Let us assume
on 5-sets with a stabilizer of order 5.
Let us assume ![]() to be
a
to be
a ![]() -
-![]() design admitting
design admitting
![]() . Then an orbit
. Then an orbit ![]() of
of ![]() of size 5 is contained in exactly one
block
of size 5 is contained in exactly one
block ![]() of
of ![]() . This block is of size 6 and has to be invariant under
. This block is of size 6 and has to be invariant under ![]() .
So,
.
So, ![]() consists of
consists of ![]() and an additional fixed point of
and an additional fixed point of ![]() .
We have to choose one of the two fixed points of
.
We have to choose one of the two fixed points of ![]() to determine the first orbit
to determine the first orbit ![]() of
of ![]() that has to belong to the design
that has to belong to the design ![]() . The two choices lead to isomorphic solutions,
since
. The two choices lead to isomorphic solutions,
since ![]() maps one of the orbits onto the other one.
maps one of the orbits onto the other one.
We now investigate other orbits of 5-sets and 6-sets.
Theorem 5:
Let
![]() and
and ![]() a 5-set whose stabilizer
a 5-set whose stabilizer
![]() in
in ![]() has an order different from 5. Then
has an order different from 5. Then
![]() For the block
For the block ![]() containing
containing ![]() of a
of a ![]() -
-![]() design admitting
design admitting
![]() we have that
we have that
![]() divides
divides ![]() .
.
Proof.
A subgroup ![]() leaving a 5-set
leaving a 5-set ![]() invariant acts on
invariant acts on ![]() .
No elements of
.
No elements of ![]() different from the identity
has more than 2 fixed points. So, the orbits on
different from the identity
has more than 2 fixed points. So, the orbits on ![]() must
be of type (5), (4,1), (3,2), (3,1,1), (2,2,1).
By assumption (5) does not occur.
Since 2 does not divide
must
be of type (5), (4,1), (3,2), (3,1,1), (2,2,1).
By assumption (5) does not occur.
Since 2 does not divide ![]() , no element of order 2 of
, no element of order 2 of ![]() has any fixed point. So, (4,1), (2,2,1) do nor occur.
By Corollary 3, no element of order 3 fixes
has any fixed point. So, (4,1), (2,2,1) do nor occur.
By Corollary 3, no element of order 3 fixes ![]() . Therefore,
. Therefore,
![]() and
and ![]() do not occur.
So,
do not occur.
So,
![]() The stabilizer
The stabilizer
![]() of a 6-set
of a 6-set ![]() then has orbits of length
then has orbits of length
![]() on the 5-sets contained in
on the 5-sets contained in ![]() .
So,
.
So,
![]() where
where ![]() is the number of orbits.
is the number of orbits.
![]()
We conclude that all stabilizers of blocks of a Steiner
5-system ![]() -
-![]() with automorphism group
with automorphism group ![]() must have orders in
must have orders in ![]() .
.
We want to derive some conditions on the numbers of blocks
which lie in orbits of a fixed length.
Let ![]() be the number of orbits of size
be the number of orbits of size ![]() of
of ![]() on the blocks of the design. Then
on the blocks of the design. Then
From the general formula for the number of blocks in a ![]() -design we
obtain in this case
-design we
obtain in this case

After cancelling we obtain
This equation can be reduced modulo some primes to get short relations.
Reducing modulo 5 gives our already known result that ![]() if
5 divides
if
5 divides ![]() because also
because also ![]() .
.
We consider possibilities of prescribing certain choices of stabilizers.
If only one of all ![]() is assumed to be non-zero then we obtain
the following results.
is assumed to be non-zero then we obtain
the following results.
Theorem 6
Let a ![]() -
-![]() design with automorphism group
design with automorphism group ![]() partition
into
partition
into ![]()
![]() -
-
![]() designs with automorphism group
designs with automorphism group ![]() consisting of only one orbit of
consisting of only one orbit of ![]() of length
of length
![]() each.
Then either
each.
Then either ![]() and
and
![]() or
or
![]() and
and
![]() or
or ![]() and
and ![]() .
.
Proof.
We only have to consider the cases
![]() .
The case
.
The case ![]() is already contained in [5]. So let now
is already contained in [5]. So let now ![]() .
Then
.
Then

One can discuss further more complicated cases of selecting several stabilizers with the same methods. We will give some experimental results on existing Steiner systems in such situations below.