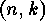 -codes
obviously do not reach as far as the enumerative methods, but the use of
computers allows to get a complete overview of linear
-codes
obviously do not reach as far as the enumerative methods, but the use of
computers allows to get a complete overview of linear 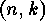 -codes over
-codes over
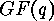 for quite a number of parameter triples
for quite a number of parameter triples 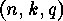 . We have seen
before that the isometry classes can be described as orbits of
. We have seen
before that the isometry classes can be described as orbits of 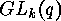 on
sets of mappings into the projective space. A very interesting and helpful
constructive method for discrete structures which can be defined as orbits of
finite groups on finite sets is based on the following fact. If
on
sets of mappings into the projective space. A very interesting and helpful
constructive method for discrete structures which can be defined as orbits of
finite groups on finite sets is based on the following fact. If 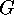 is a
transitive permutation group on
is a
transitive permutation group on 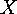 , then the orbits of a subgroup
, then the orbits of a subgroup 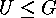 on
on 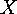 can be bijectively mapped onto double cosets as follows: For any
can be bijectively mapped onto double cosets as follows: For any 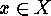 the mapping
the mapping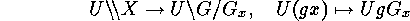
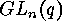 is transitive on the set
is transitive on the set 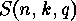 of
subspaces of dimension
of
subspaces of dimension 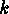 in
in 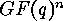 , so that the isometry classes of
linear
, so that the isometry classes of
linear 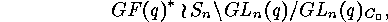
 is any linear
is any linear